
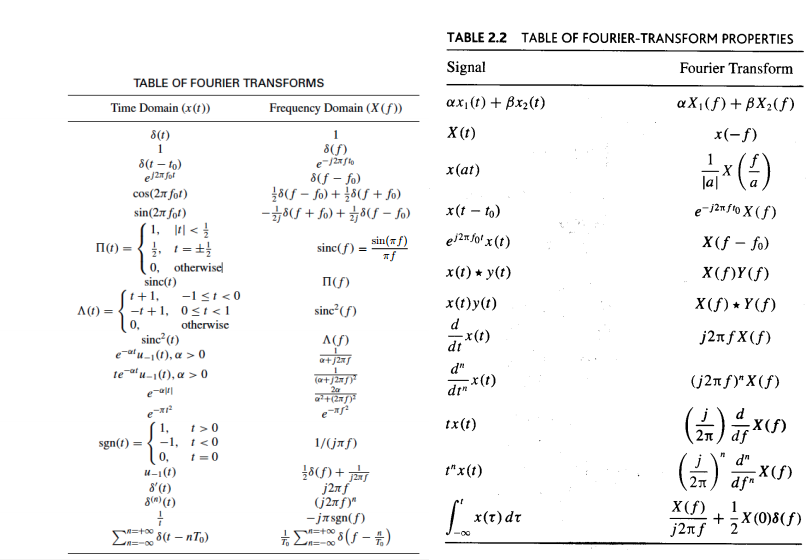
It should be useful for everyone concerned with Fourier. To switch to different definitions would just add confusion. This book presents a comprehensive collection of integrals of the Fourier transformation type. When the magnitude spectrum is positive, then the phase is zero and if the magnitude spectrum is negative, then the phase is $(±\pi)$. Wikipedias tables list Fourier transform pairs for three different versions of the Fourier transform and it is not surprising that the same definition of sinc is used in the table. The phase spectrum of the rectangular function is an odd function of the frequency (ω). The main lobe becomes narrower with the increase in the width of the rectangular pulse. $$\mathrm\right)$are known as the side lobes.įrom the magnitude spectrum, it is clear that the majority of the energy of the signal is contained in the main lobe. Table of Fourier Transform Pairs Function, f(t) Fourier Transform, F(w) Definition of Inverse Fourier Transform Definition of Fourier Transform 1 F (w ). The term discrete-time refers to the fact that the transform operates on discrete data, often samples whose interval has units of time. First fundamental frequency (left) and original waveform (right) compared. c and s are parameters of the Fourier transform. The first component is a sinusoidal wave with period T6.28 (2pi) and amplitude 0.3, as shown in Figure 1. The Fourier transform of the expression f f(x) with respect to the variable x at the point w is.

#FOURIER TRANSFORM TABLE SERIES#
The DTFT is often used to analyze samples of a continuous function. This decomposition can be done with a Fourier transform (or Fourier series for periodic waveforms), as we will see. I also checked the book "Table of Integrals, Series, and Products" by Gradshteyn and Ryzhik, and couldn't find the thing I'm looking for.The Fourier transform of a continuous-time function $x(t)$ can be defined as, In mathematics, the discrete-time Fourier transform (DTFT) is a form of Fourier analysis that is applicable to a sequence of values. Table of Fourier Transform Pairs Function, f(t) Fourier Transform, F(w) Definition of Inverse Fourier Transform Definition of Fourier Transform Signals &. The function $f(\xi)=(i-\xi)^a\cdot(\log(i-\xi))^b$ can be defined for all real $\xi$ (by choosing appropriate branches of $\log(i-\xi)$ and $\log(\log(i-\xi))$), and the inverse Fourier transform of $f(\xi)$ makes sense as a distribution on $\mathbb$ As was commented below, the Erdelyi book "Tables of Integral Transforms" is the same as the one I referred to above when I mentioned the Bateman project. Recall the definition of hyperbolic functions. The result of FFT doesnt match analytic transform in most cases by. The fast Fourier transformation1 (FFT) is widely used in many digital signal processing applications in both the frequency and time domain. This list is not a complete listing of Laplace transforms and only contains some of the more commonly used Laplace transforms and formulas. The point is that a normal polynomial multiplication requires O ( N 2 ) O(N2) O ( N 2 ) multiplications of integers, while the coordinatewise multiplication in this. Dear friends, I done FFTs of good known functions from Fourier Transform pairs table. Note that I failed to find the answer not only online, but also in the standard books with Fourier transform tables (such as "Tables of Integral Transforms" from the Bateman project). This may seem like a roundabout way to accomplish a simple polynomial multiplication, but in fact it is quite efficient due to the existence of a fast Fourier transform (FFT).

My question is in fact motivated by one concrete example (so if you know the answer to this one, please let me know!). In this video, we learn about Fourier transform tables which enable us to quickly travel from time to the frequency domain. I was wondering if someone can suggest a website (or some online document) containing an $extensive$ table of Fourier transforms? When I try obvious Google searches, like "table of Fourier transforms", the several dozen top results give extremely short tables.


 0 kommentar(er)
0 kommentar(er)
